SM0486
difference of the two volumes) and 0.0012 gm/cm3 (the density of air), we determine
the value (in terms of mass) of the error caused by the buoyancy of air is
k. We could also figure the buoyant effect air has on each of the two
different masses and then subtract to find the difference in buoyant effect. Since
the brass standard had the greatest volume, we can state that air had the greatest
effect on it.
From the preceding example since the brass was our standard, we
should add the mass correction due to buoyancy to the stainless steel mass.
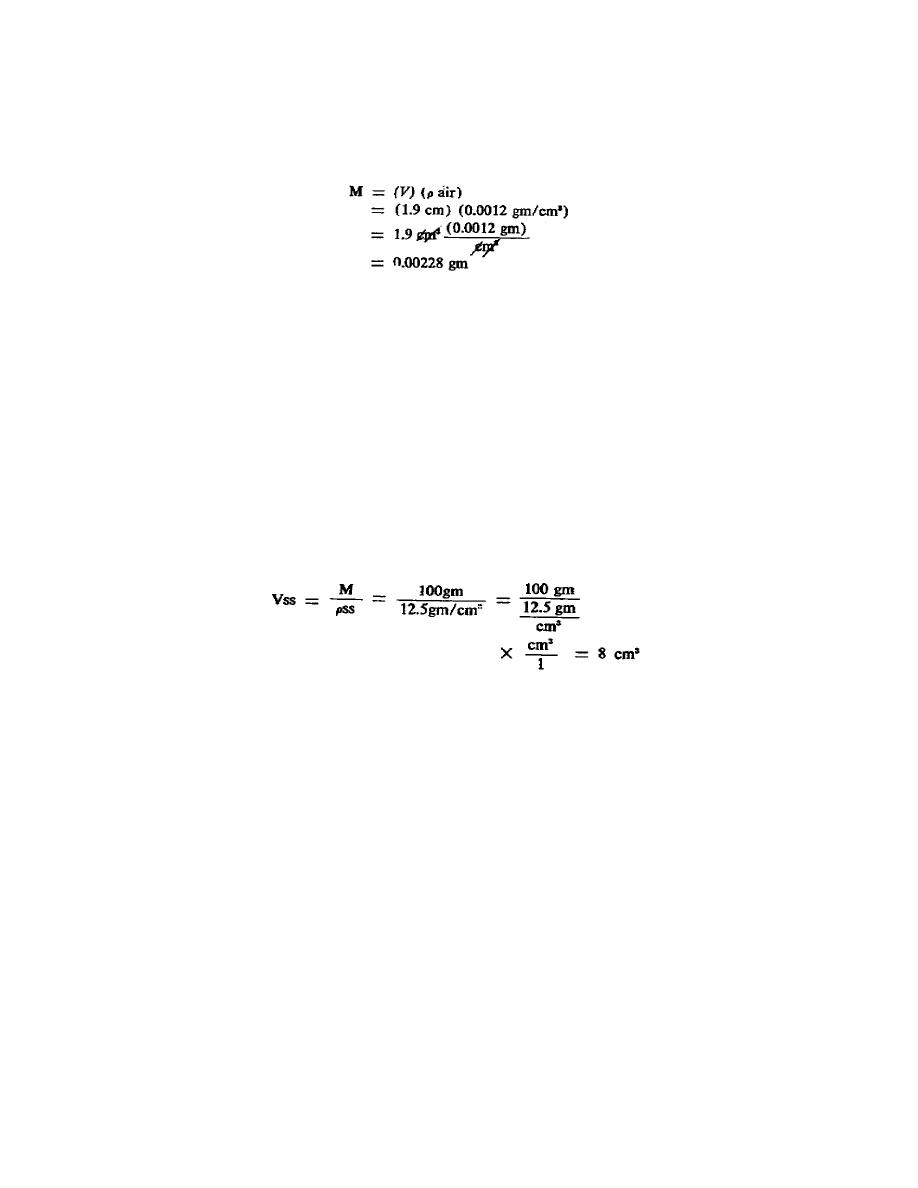
l. Now, using the information and examples, determine the buoyancy correction
for the 100-gram stainless steel mass in the example after we arbitrarily change
its density from 10 grams per cm3 to 12.5 grams per cm3. Use the questions which
follow as a guide to your solution:
(1) What value or values do you need?
Examine the example.
The volumes of the
unknown and the standard were computed.
Since brass is our standard in this
problem, its volume is the same. For the stainless steel mass with the arbitrary
density of 12.5 grams per cm3 its volume becomes:
(2) What is the second step, after the volumes for stainless steel and
brass are known? As stated before, one easy method of finding an equivalent mass
for buoyancy is to find the difference between the two masses and then find the
product of the calculated differences and the density of air, (ΔV) (). This means
that your next step is to determine the differences in volume for the 100-gram mass
standard brass and the 100-gram mass of stainless steel.
(3) You know the difference between the two volumes.
What next?
137


 Previous Page
Previous Page
